Completing the Square
Completing the square is a process that helps us rewrite a quadratic that's in the form ax2 + bx + c into the form a(x - h)2+k. So, why would we want to do this? Well, for starters, if you want to graph the parabola, a(x - h)2+k is a form that's easier to work with because you automatically know that the vertex is at (h, k).
(Also, if you're taking calculus, then knowing how to complete the square may help you integrate a complicated function; or, if you're taking a course in differential equations, it may help you to find the transformation of a function).
How to Complete the Square
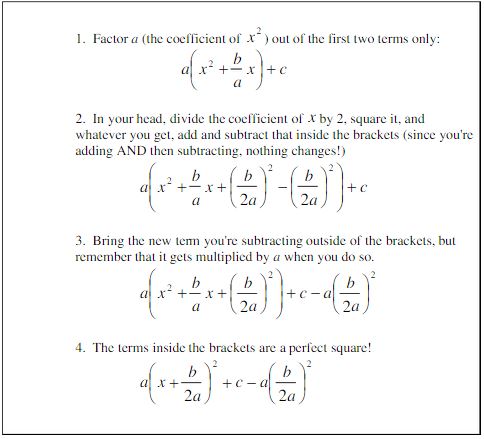
Starting with ax2 + bx + c, here's how to complete the square:
OK, it's quite tricky to make sense of this just by looking at the steps, so hopefully the examples should make a lot more sense!
Example: Complete the square to write x2 + 6x + 11 in the form of a(x - h)2+k.
Solution:
x2 + 6x + 11
=(x2 + 6x + 9 - 9) + 11
=(x2 + 6x + 9) + 11 - 9
=(x2 + 6x + 9) + 2
= (x + 3)2 + 2
Example: Complete the square to write 2x2 - 16x + 10 in the form a(x - h)2+k.
2x2 - 16x + 10
= 2(x2 - 8x) + 10
= 2(x2 - 8x + 16 - 16) + 10
= 2(x2 - 8x + 16) -32 + 10
= 2(x - 4)2 -22
An Important Note:
Remember that if you expand the expression that you get for your final answer, you'll get the original expression in the question...this is a useful way to check your work!
Example 1:
Example 2:
Example 3: