Transformations of Trigonometric Functions
A QUICK REVIEW
The transformation of functions includes the shifting, stretching, and reflecting of their graph. The same rules apply when transforming trigonometric functions.
VERTICAL AND HORIZONTAL SHIFTS
Suppose \(c > 0\). To obtain the graph of
\( y = f(x) + c\) : shift the graph of \( y = f(x)\) up by \(c\) units;
\( y = f(x) - c\) : shift the graph of \( y = f(x)\) down by \(c\) units;
\( y = f(x - c) \): shift the graph of \( y = f(x)\) to the right by \(c\) units;
\( y = f(x + c) \): shift the graph of \( y = f(x)\) to the left by \(c\) units;
Example: Sketch the functions \(y = \sin(x)\), \(y = \sin(x) +4 \), and \(y = \sin(x + \frac{\pi}{2})\)
Solution:
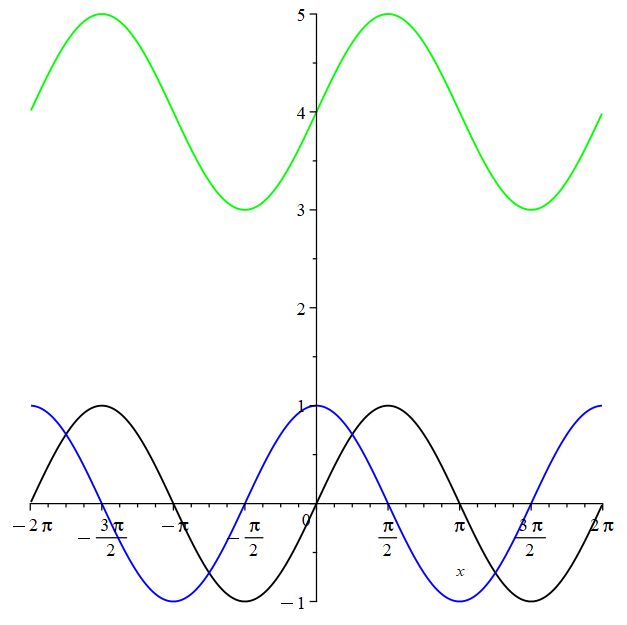
Here, \( y = \sin(x)\) is shown in black. The curve \( y = \sin(x) + 4\) is shifted 4 units up, shown in green. The curve \(y = \sin(x + \frac{\pi}{2})\) is shifted \(\frac{\pi}{2}\) units to the left and is shown in blue. Notice this is the same thing as the curve \( y = \cos(x)\).
VERTICAL AND HORIZONTAL STRETCHES/COMPRESSIONS
Suppose \(c > 1\). To obtain the graph of
\( y = cf(x)\): stretch the graph of \( y =f(x)\) vertically by a factor of \(c\);
\( y = \frac{1}{c}f(x)\): compress the graph of \( y =f(x)\) vertically by a factor of \(c\);
\( y = f(cx)\): compress the graph of \( y =f(x)\) horizontally by a factor of \(c\);
\( y = f(\frac{1}{c}x)\): stretch the graph of \( y =f(x)\) horizontally by a factor of \(c\);
Example: Sketch the functions \(y = \cos(x)\), \(y = \cos(2x)\), and \(y = 3\cos(2x)\).
Solution:
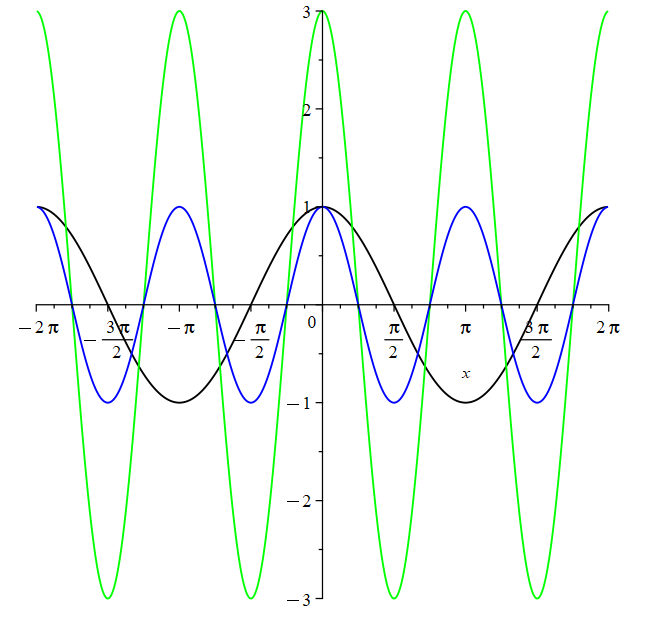
The function \(y = \cos(x)\) is shown in black. The blue curve represents the function \(y = \cos(2x)\) and is compressed horizontally by a factor of 2. The green curve represents the function \(y = 3\cos(2x)\) and is the blue curve stretched vertically by a factor of 3.
REFLECTIONS
To obtain the graph of
\(y = -f(x)\): reflect the graph of \(y = f(x) \) about the \(x\)-axis; and
\(y = f(-x)\): reflect the graph of \( y = f(x) \) about the \(y\) - axis
Example:
Given that the blue curve represents the function \( y = \sin(x) \), determine whether the following statement is TRUE or FALSE.
The green curve on the following graph represents both \( y = -\sin(x)\) and \( y = \sin(-x) \).

