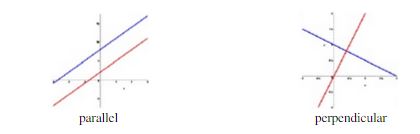Introduction to Linear Functions

The concept of slope

A linear function is simply the mathematical way of representing a straight line on the xy-plane. One important aspect of the line is its steepness or slope, typically denoted m. The slope of a line is defined as the vertical change (the “rise”) over the horizontal change (the “run”) as one travels alone the line.

Therefore, the slope of a vertical line does not exist because it does not satisfy the above restriction. In the case of a horizontal line, the slope does exist and have a value of 0.

Parallel and Perpendicular Lines

When working with linear equations we will most frequently use the form y = mx + b for the equation of a line, where m is the slope. Sometimes, when working with a given line we are also interested in lines which are parallel or perpendicular to the particular line.

• Two lines are parallel if there slopes are the same (or both are vertical).
• Two lines are perpendicular if the product of their slopes, m₁m₂ = -1 (or if one line is vertical while the other is horizontal).

Example: Are y = 4x + 3 and 3y – 12x + 9 = 0 parallel lines?

Solution: The slope of the first line is 4. In order to be parallel, both lines must have the same slope. Therefore, we must rearrange the second equation to the form y = mx + b to determine if it has the same slope as the first line.

3y – 12x + 9 = 0

                 -3y = -12x + 9

                     y = 4x – 3

Both lines have the same slope m₁ = m₂ = 4, therefore, they are parallel lines. 

Example: Are the lines y = 3x + 7 and y = 1/3x + 4 perpendicular?

Solution:

m₁m₂ = 3 ∙ 1/3

           = 1

Since m₁m₂ ≠ -1, therefore, the two lines are not perpendicular

Example: Given y = 5x + 2, find the slope for the perpendicular line.

Solution: We want m₁m₂ = -1 

m₁m₂ = -1 

   5m₂  = -1

      m₂ = - 1/5